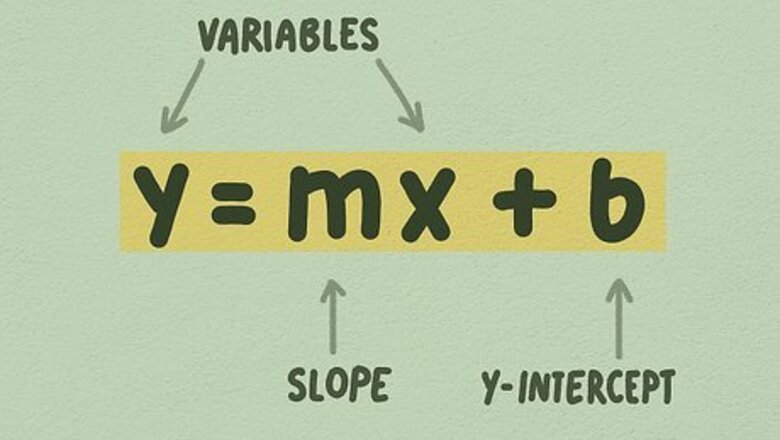
views
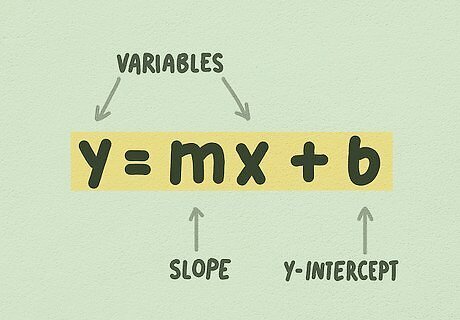
Make sure the linear equation is in the form y = mx + b. This is called the y-intercept form, and it's probably the easiest form to use to graph linear equations. The values in the equation do not need to be whole numbers. Often you'll see an equation that looks like this: y = 1/4x + 5, where 1/4 is m and 5 is b. m is called the "slope," or sometimes "gradient." Slope is defined as rise over run, or the change in y over the change in x. b is defined as the "y-intercept." The y-intercept is the point at which the line crosses the Y-axis. x and y are both variables. You can solve for a specific value of x, for example, if you have a y point and know the m and b values. x, however, is never merely one value: its value changes as you go up or down the line.
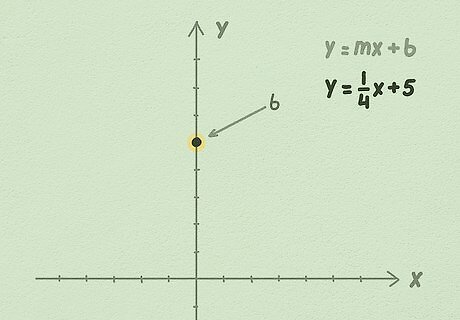
Plot the b number on the Y-axis. Your b is always going to be a rational number. Just whatever number b is, find its equivalent on the Y-axis, and put the number on that spot on the vertical axis. For example, let's take the equation y = 1/4x + 5. Since the last number is b, we know that b equals 5. Go 5 points up on the Y-axis and mark the point. This is where your straight line will pass through the Y-axis.
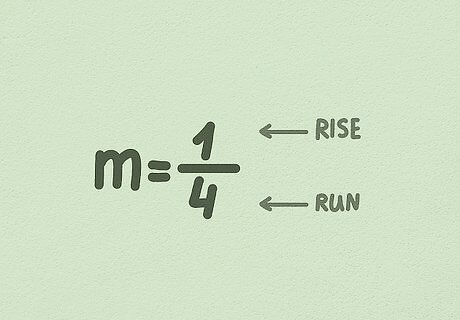
Convert m into a fraction. Often, the number in front of x is already a fraction, so you won't have to convert it. But if it isn't, convert it by simply placing the value of m over 1. The first number (numerator) is the rise in rise over run. It's how far the line travels up, or vertically. The second number (denominator) is the run in rise over run. It's how far the line travels to the side, or horizontally. For example: A 4/1 slope travels 4 points up for every 1 point over. A -2/1 slope travels 2 points down for every 1 point over. A 1/5 slope travels 1 point up for every 5 points over.
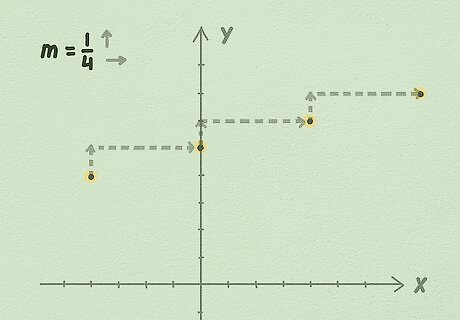
Start extending the line from b using slope, or rise over run. Start at your b value: we know that the equation passes through this point. Extend the line by taking your slope and using its values to get points on the equation. For example, using the illustration above, you can see that for every 1 point the line rises up, it travels 4 to the right. That's because the slope of the line is 1/4. You extend the line indefinitely along both sides, continuing to use rise over run to graph the line. Whereas positive-value slopes travel upward, negative-value slopes travel downward. A slope of -1/4, for example, would travel down 1 point for every 4 points it travels to the right.
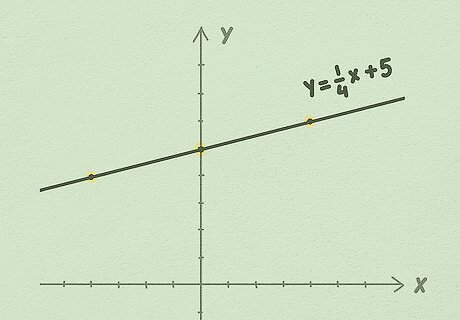
Continue extending the line, using a ruler and being sure to use the slope, m, as a guide. Extend the line indefinitely and you're done graphing your linear equation. Pretty easy, isn't it? EXPERT TIP Joseph Meyer Joseph Meyer Math Teacher Joseph Meyer is a High School Math Teacher based in Pittsburgh, Pennsylvania. He is an educator at City Charter High School, where he has been teaching for over 7 years. Joseph is also the founder of Sandbox Math, an online learning community dedicated to helping students succeed in Algebra. His site is set apart by its focus on fostering genuine comprehension through step-by-step understanding (instead of just getting the correct final answer), enabling learners to identify and overcome misunderstandings and confidently take on any test they face. He received his MA in Physics from Case Western Reserve University and his BA in Physics from Baldwin Wallace University. Joseph Meyer Joseph Meyer Math Teacher Develop strong graphing skills. Drawing graphs by hand will help you develop foundational graphing skills, especially in understanding scales and axes. This will build a strong base for you to use helpful online tools to visualize complex relationships, perform calculations, and prepare for standardized tests.

















Comments
0 comment